Multiple Slit Diffraction for Engineering Physics B.Tech 1st Year

What is Diffraction?

Diffraction is when waves like light or sound spread out as they move around an object or through a slit. When light passes through each of the slits, it will spread out and overlap with the light from the other slit. It’s through this overlapping that the diffraction pattern of dark and bright areas is created.

Diffraction due to N-Slits (Grating):

An arrangement consisting of large number of parallel slits of the same width and separated by equal opaque spaces is known as Diffraction grating.Gratings are constructed by ruling equidistant parallel lines on a transparent material such as glass, with a fine diamond point. The ruled lines are opaque to light while the space between any two lines is transparent to light and acts as a slit. This is known as Gratings are constructed by ruling equidistant parallel lines on a transparent material such as glass, with a fine diamond point. The ruled lines are opaque to light while the space between any two lines is transparent to light and acts as a slit. This is known as plane transmission grating. When the spacing between the lines is of the order of the wavelength of light, then an appreciable deviation of the light is produced.

Mathematical Expression:

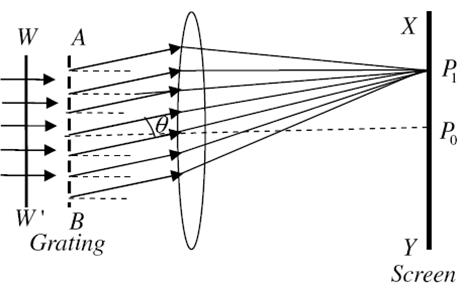
Let ‘e’ be the width of each slit and ‘d’ the width of each opaque space. Then (e+d) is known as grating element and XY is the screen. Suppose a parallel beam of monochromatic light of wavelength ‘ ‘ be incident normally on the grating. By Huygen’s principle, each of the slit sends secondary wavelets in all directions. Now, the secondary wavelets traveling in the direction of incident light will focus at a point P_o on the screen. This point P_o will be a central maximum.

Now consider the secondary waves traveling in a direction inclined at an angle ‘ ‘ with the incident light will reach point P₁ in different phases. As a result dark and bright bands on both sides of central maximum are obtained.

The intensity at point P₁ may be considered by applying the theory of Fraunhofer diffraction at a single slit. The wavelets proceeding from all points in a slit along their direction are equivalent to a single wave of amplitude starting from the middle point of the slit, Where

If there are N slits, then we have N diffracted waves. The path difference between two consecutive slits is

Therefore, the phase difference

Hence the intensity in a direction ‘v ‘ can be found by finding the resultant amplitude of N vibrations each of amplitude   and a phase difference of ‘ ‘

Since in the previous case

Substituting these in equation

The resultant amplitude on screen at P₁ becomes

Thus Intensity at P₁ will be

The factor

 

 

gives the distribution of Intensity due to a single slit while the factor

gives the distribution of Intensity due to a single slit while the factor

gives the distribution of intensity as a combined effect of all the slits.

Intensity Distribution:

Case (i): Principal maxima:

The eqn (2.40) will take a maximum value if

………(2.42)

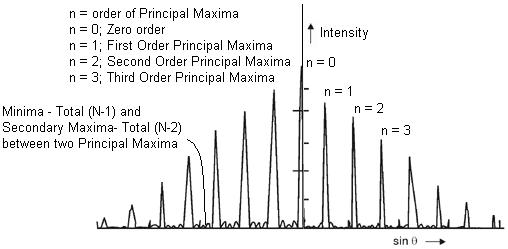
n = 0 corresponds to zero order maximum. For n = 1,2,3,… we obtain first, second, third,… principal maxima respectively. The ± sign indicates that there are two principal maxima of the same order lying on either side of zero order maximum.

Case(ii): Minima Positions:

The eqn (2.40) takes minimum value if but


………..(2.43)

Where m has all integral values except m = 0, N, 2N, …, nN, because for these values  becomes zero and we get principal maxima. Thus, m = 1, 2, 3, …, (N-1). Hence

where

gives the minima positions which are adjacent to the principal maxima.

…………(2.44)

The roots of the above equation other than those for which give the positions of secondary maxima The eqn (2.44) can be written as

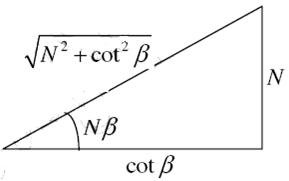
From the triangle we have

Since intensity of principal maxima is proportional to N²

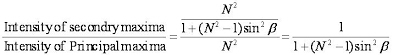
Hence if the value of N is larger, then the secondary maxima will be weaker and becomes negligible when N becomes infinity

Feedback is important to us.