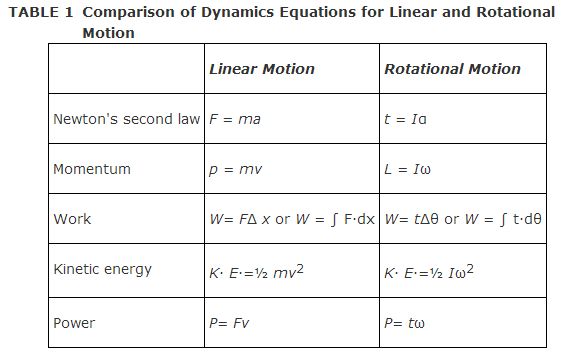
Rotational Motion of a Rigid Body:
Rotational motion is more complicated than linear motion, and only the motion of rigid bodies will be considered here. A rigid body is an object with a mass that holds a rigid shape, such as a phonograph turntable, in contrast to the sun, which is a ball of gas. Many of the equations for the mechanics of rotating objects are similar to the motion equations for linear motion.
Angular Velocity and Angular Acceleration:
The angular displacement of a rotating wheel is the angle between the radius at the beginning and the end of a given time interval. The SI units are radians. The average angular velocity (ω, Greek letter omega), measured in radians per second, is
![]()
The angular acceleration (α, Greek letter alpha) has the same form as the linear quantity
![]()
and is measured in radians/second/second or rad/s 2.
The kinematics equations for rotational motion at constant angular acceleration are
Consider a wheel rolling without slipping in a straight line. The forward displacement of the wheel is equal to the linear displacement of a point fixed on the rim. As can be shown in Figure , d = S = rθ
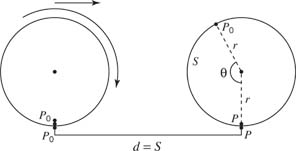
In this case, the average forward speed of the wheel is
v = d/ t = ( rθ)/ t = rω,
where r is the distance from the center of rotation to the point of the calculated velocity.
The direction of the velocity is tangent to the path of the point of rotation.
The average forward acceleration of the wheel is
a T = r(ω f − ω o)/ t = rα.
This component of the acceleration is tangential to the point of rotation and represents the changing speed of the object. The direction is the same as the velocity vector.
The radial component of the linear acceleration is
a r = v 2/ r = ω 2 r.
Torque:
It is easier to open a door by pushing on the edge farthest from the hinges than by pushing in the middle. It is intuitive that the magnitude of the force applied and the distance from the point of application to the hinge affect the tendency of the door to rotate. This physical quantity, torque, is
t = r × F sin θ,
where F is the force applied, r is the distance from the point of application to the center of the rotation, and θ is the angle from r to F.
Moment of Inertia:
Substitute Newton’s second law into the definition for torque with θ of 90 degrees (a right angle between F and r) and use the relationship between linear acceleration and tangential angular acceleration to obtain
t = r F = rma = mr 2 ( a/ r) = mr 2α.
The quantity mr 2 is defined as moment of inertia of a point mass about the center of rotation.
Imagine two objects of the same mass with different distribution of that mass. The first object might be a heavy ring supported by struts on an axle like a flywheel. The second object could have its mass close to the central axis. Even though the masses of the two objects are equal, it is intuitive that the flywheel will be more difficult to push to a high number of revolutions per second because not only the amount of mass but also the distribution of the mass affects the ease in initiating rotation for a rigid body.
The general definition of moment of inertia, also called rotational inertia, for a rigid body is
I = ∑ m i r i 2
and is measured in SI units of kilogram‐meters 2.
The moments of inertia for different regular shapes are shown in Figure 2.

Mechanics problems frequently include both linear and rotation motions.
Example 1: Consider Figure 3, where a mass is hanging from a rope wrapped around a pulley. The falling mass (m) causes the pulley to rotate, and it is no longer necessary to require the pulley to be massless. Assign mass ( M) to the pulley and treat it as a rotating disc with radius (R). What is the acceleration of the falling mass, and what is the tension of the rope?
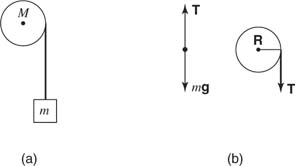
The force equation for the falling mass is
T − mg = − ma.
The tension of the rope is the applied force to the edge of the pulley that is causing it to rotate. Thus,
t = Iα, or TR = (1/2) MR 2( a/R),
which reduces to T = (1/2) Ma,
where angular acceleration has been replaced by a/R because the cord does not slip and the linear acceleration of the block is equal to the linear acceleration of the rim of the disk. Combining the first and last equation in this example leads to
Solution:
![]()
Angular momentum is rotational momentum that is conserved in the same way that linear momentum is conserved. For a rigid body, the angular momentum (L) is the product of the moment of inertia and the angular velocity: L = Iω.
For a point of mass, angular momentum can be expressed as the product of linear momentum and the radius ( r): L = mvr.
L is measured in units of kilograms‐meters 2 per second or more commonly joule‐seconds.
The law of conservation of angular momentum can be stated that the angular momentum of a system of objects is conserved if there is not external net torque acting on the system.
Analogous to Newton’s law (F = Δ( mv)/Δ t)
there is a rotational counterpart for rotational motion: t = Δ L/Δ t,
or torque is the rate of change of angular momentum.
Rotational Kinetic Energy, Work, and Power:
Kinetic energy, work, and power are defined in rotational terms as
K. E=(1/2) Iω 2, W= tθ, P= tω.
Comparison of Dynamics Equation for Linear and Rotational Motion:
The dynamic relations are given to compare the equation for linear and rotational motion (see Table ).